=================================================================================
Bethe [1] in 1930 derived the expression for the inner-shell
ionization cross-section from the first Born
approximation. As a result, it is only expected to be
valid if the incident ionizing electron energy is suficiently
high, e.g. in TEM and SEM conditions. The total ionization cross section derived by Bethe (called Bethe cross section) can be given by,
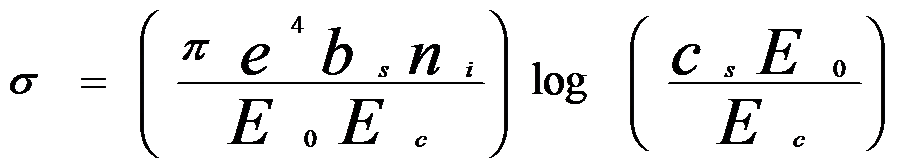 -------- [4791a] -------- [4791a]
ni – The number of electrons in a shell or subshell (e.g., ni = 2 for a K-shell, ni = 8 for an L-shell, and ni = 18 for an M-shell
bs, c s – The constants for a particular inner-shell and from least-squares fitting to ionization data
Ec – Ionization energy of given element A in the K-, L-, or M-shell (keV)
E0 -- Accelerating voltage of the incident electrons.
Reducing the acceleration voltage increases the ionization cross-section, which is determined by the overvoltage ratio. If we apply overvoltage into Equation [4791a], and then, we can have,
 -------- [4791b] -------- [4791b]
Here, the dimensions are ionizations/e-/(atom/cm2).
In low-voltage electron microscopes, e.g. low-voltage SEMs, the overvoltage can affect the generation of X-rays because the ionization cross section depends on the value of overvoltage. The optimum Ui for the ionization of the i shell (i shell is K-, L, M- shell for a characteristic x-ray) is 3~5 as indicated in Figure 4791. Then, the cross section decreases at overvoltages lower than ~30. In the TEM E0 is ≥ 100 keV and Ei is generally < 20 keV and thus, U is usually > 5. Therefore, the cross section is pretty constant with energy.
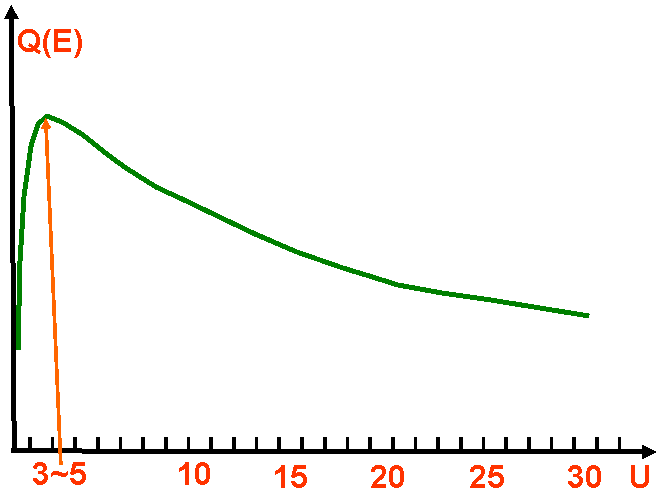
| Figure 4791. Plot of the ionization cross-section over U. Ionization is most probable if the beam energy is 3~5 of the critical ionization energy. |
Generally EELS (Electron Energy Loss Spectroscopy) has better sensitivity than EDS (Energy-dispersive X-ray spectroscopy), due to the potential of utilizing signals
generated from larger ionization cross sections (L rather than K) and the much greater collection
efficiency. [2 - 3]
[1] Bethe, HA 1930 Zur Theorie des Durchgangs Schneller Korpuskularstrahlen Durch Materie Ann. der Phys.
Leipzig 5 325–400.
[2] M. Isaacson, D. Johnson, Ultramicroscopy 1 (1975) 33.
[3] H. Shuman, P. Kruit, Rev. Sci. Instrument 56 (1985) 231.
|