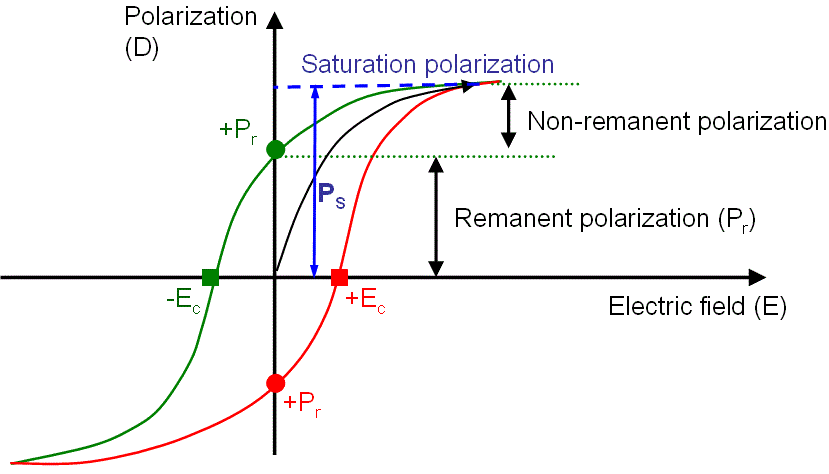
Dielectric hysteresis was discovered by Valasek [1] and may be defined as an effect in a dielectric material similar to the hysteresis found in magnetic materials. In this case, the electric displacement D for one direction in the crystal (parallel to the x axis) was not only determined by the applied field E, but also depended on its previous values.

A typical hysteresis loop is shown in Figure 1804a. At low electric fields and at very high electric fields a ferroelectric behaves like an ordinary dielectric with a high dielectric constant, but at the coercive field E_c polarization reversal occurs and induces a large dielectric non-linearity. At zero field (E₀) the electric displacement within a single domain has two values (-P_r and +P_r), representing the opposite orientations of the spontaneous polarization. In a multiple-domain crystal the average zero-field displacement can have any value between these two extremes (-P_r < D < +P_r).

Figure 1804a. A typical dielectric hysteresis loop (E_c: coercive field; P_s: spontaneous polarization; and P_r: remanent polarization).

Different from ferroelectric hysteresis, the schematic illustrations in Figure 1804b show D-E loops of four non-ferroelectric dielectrics.

	Lossless	Lossy
Linear	(a)	(b)
Non-linear	(c)	(d)

Figure 1804b. D-E loops of four non-ferroelectric dielectrics: (a) Linear lossless dielectric, (b) Linear lossy dielectric, (c) Non-linear lossless dielectric, and (d) Non-linear lossy dielectric.