=================================================================================
According to the theory of Turnbull and Cohen, [7] certain open volume or space in glass-forming liquids exist around each atom. When this volume reaches a critical value, less energy is needed to move the atom in and out of it, making possible diffusion and flow. Such a space or volume is called “free volume” (FV). For instance, Liu et al. [8] proposed that the atomic structure of Zr2Ni metallic glass is essentially an association of ordered clusters and FV. The ordered clusters in size of ~ 1.5 nm consist of a densely packed core (i.e. icosahedral or fcc-type packing) and are surrounded loosely by large FV.
The method based on FFT and inverse FFT developed by Miller and Gibson [2] and extended by Li et al. [3] and Jiang and Atzmon [4] later was applied to analyze the SRO (short range ordering) clusters by Zhu et al. [1]. They employed a core–shell model to investigate the shear bands in as-cast Zr64Nb6Cu13.5Ni8.5Al8 specimen (Figure 1698). In Figure 1698 (b), the bright-yellow part represents the zone with more free volume
(FV), while the dark-blue part represents the zone with less FV. The core is proposed as SRO clusters with a different coordination number (CN) [5] and the local FV is shell. It is defined that SRO clusters with CN of 12 or more than 12 have no FV, while ordering clusters with CN < 12 have certain FV [6].
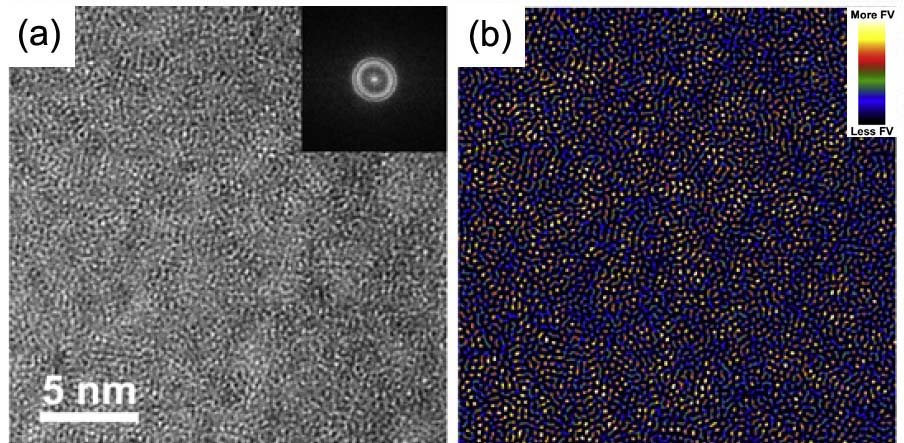
Figure 1698. (a) HRTEM image of an as-cast sample for Zr64Nb6Cu13.5Ni8.5Al8 alloys (the inset presents fast Fourier transform (FFT) pattern), and (b) The corresponding Fourier-filtered, threshold filtered and inverted image. Adapted from [1]
The concept of defects in metallic glasses is suggested in free-volume theory. In this theory, a defect is defined as a site at which the free volume exceeds a critical value that is on the order of an atomic volume.
[1] Z.W. Zhu, L. Gu, G.Q. Xie, W. Zhang, A. Inoue, H.F. Zhang, Z.Q. Hu, Relation between icosahedral short-range ordering and plastic deformation in Zr–Nb–Cu–Ni–Al bulk metallic glasses, Acta Materialia 59 (2011) 2814–2822.
[2] Peter D. Miller and J. Murray Gibson, Connecting small-angle diffraction with real-space images by quantitative transmission electron microscopy of amorphous thin-Þlms, Ultramicroscopy 74 (1998) 221-235.
[3] Li J, Wang ZL, Hufnagel TC. Phys Rev B 2002;65:144201.
[4] Jiang WH, Atzmon M. Acta Mater 2003;51:4095.
[5] Miracle DB, Sanders WS, Senkov ON. Philos Mag 2003;83:2409.
[6] Liu XJ, Chen GL, Hui XD, Liu CT, Lu ZP. Appl Phys Lett
2008;93:011911.
[7] M. H. Cohen and D. Turnbull: J. Chem. Phys. 31 (1959) 1164–1169.
[8] X. J. Liu, G. L. Chen, X. Hui, T. Liu, and Z. P. Lu, Ordered clusters and free volume in a Zr–Ni metallic glass, Appl Phys Lett, 93, 011911 (2008).
|
