=================================================================================
Based on the theory of wave aberration function and the relationship between the scattering angle and the reciprocal position u at the back focal plane of a lens, we can convert the distance W to a phase shift (also called phase difference) (see Equation 3752a). Therefore, the phase shifts due to the spherical aberration and defocus (as well as defect of focus) can be combined into a single phase factor χ, given by,
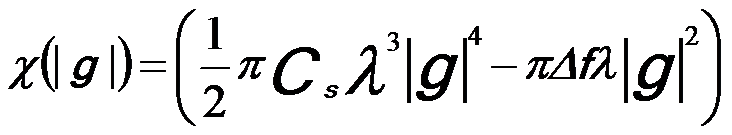 -------------- [2751a.a] -------------- [2751a.a]
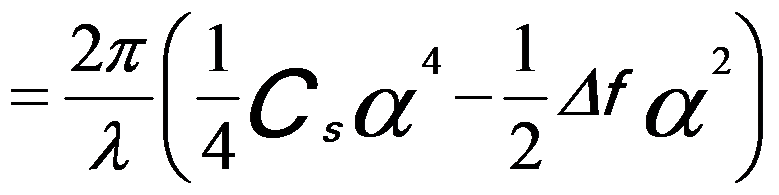 -------------- [2751a.b] -------------- [2751a.b]
where,
Cs -- Spherical aberration coefficient, defining the quality of objective lens,
λ -- Wave-length,
Δf -- Defocus value,
|g| -- Spatial frequency,
C1 = Δf,
α -- The convergence semi-angle.
The first term on the right-hand side in Equations 2751a represents the effect from the spherical aberration while the second term represents that from the defocus of the objective lens.
As indicated in Figure 2751, the spherical aberration from a round lens leads the rays off-axis to be deflected more strongly than for an ideal lens, resulting in a positive phase shift. Based on Equations 2751a, at low convergence semi-angles (α) in STEM, this phase shift caused by the spherical aberration can be partially compensated for the low spatial frequencies by applying a negative defocus, while at high α, this phase shift cannot efficiently be canceled out because the spherical aberration dominates the aberration function χ(α). In general, a maximum acceptable phase shift of π/2 (i.e. a quarter wavelength) across the objective lens is practically tolerated, allowing a maximum convergence semi-angle α0.

Figure 2751. Compensation of phase shift between spherical aberration and defocus.
|
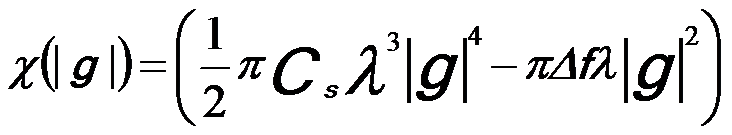 -------------- [2751a.a]
-------------- [2751a.a] 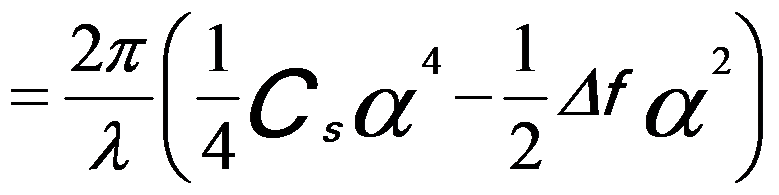 -------------- [2751a.b]
-------------- [2751a.b] 