=================================================================================
With the illumination geometry of CBED (Convergent Beam Electron Diffraction), the incident beam directions vary continuously within the cone. The continuous variation of incident beam directions induces a continuous variation of the excitation error of the Bragg reflection and the observed intensity distribution in the CBED disks corresponds to a two-dimensional rocking curve (pendellösung) of the dynamical theory of electron diffraction.
Similar to the case of “single-electron beam” tilt in TEM, with a convergent incident beam we can split the beam into many electron rays, which are smaller electron beams C1 to Cn shown in Figure 4136. Each incident ray inside the incident beam has a different Ewald sphere because it has a different ray orientation. All those Ewald spheres locate between the two extreme spheres ‘‘-α’’ and ‘‘+α’’. Differently intersecting the same relrod by different Ewald sphere gives different excitation error (s). Each reciprocal lattice point located between these two extreme spheres gives a diffracted intensity. One application of CBED is, therefore, a large convergence at a semi-angle α can be very useful to obtain a large number of reflections. The available convergent semi-angle in modern TEMs can be as high as 3 – 5°. It is important to mention that the intensity of the convergent diffraction beams is the integral (indicated by the green area in the inset) of the relrod portion located between the two extreme Ewald spheres.
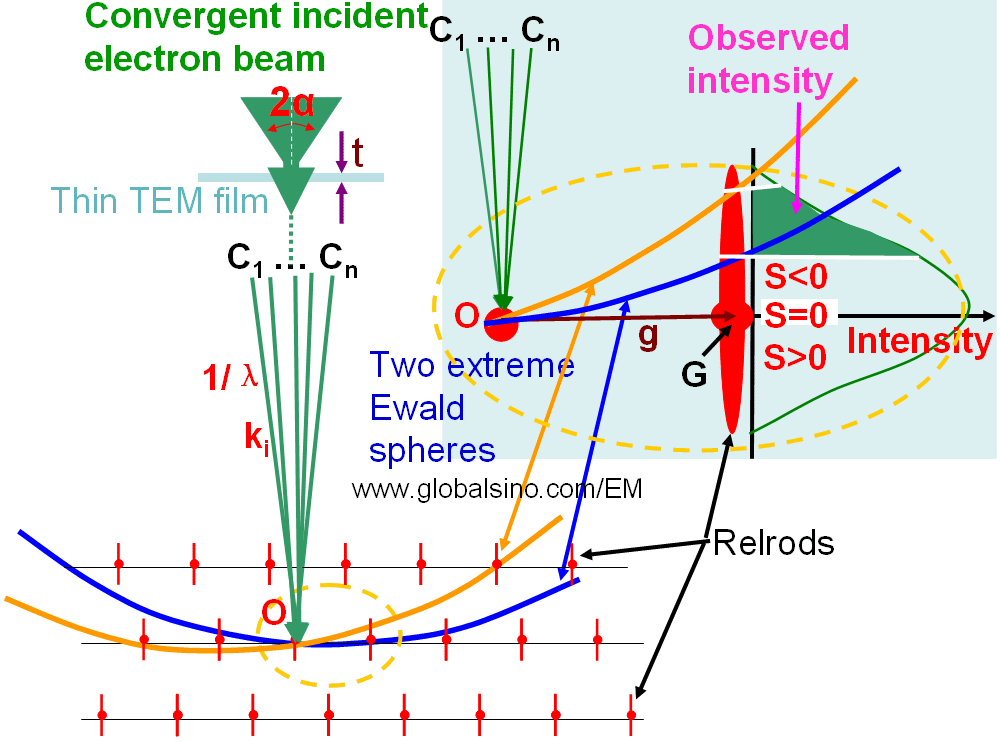
Figure 4136. Ewald sphere construction with a convergent incident electron beam on a thin TEM film.
The dotted line along the electron beam indicates the beam path through lenses.
Furthermore, the intensity distribution of CBED patterns depends also on other factors such as composition, stoichiometry and defects in the crystals and the quality of the TEM specimens.
|